Solar and Lunar Reflection Skyscraper Geometry
|
An unexpected wonderful sight creates another experience of a lifetime!
Although I had carefully chosen the location to catch the Full Moon setting above the Alberta Legislature, I was unaware of the coincidence in timing, geometry, and really good weather that was needed for a simultaneous reflection to happen. More than a decade later I still have not managed to recapture this event.
In this article I extend my discussion in the 2005 JRASC article to reflections from water, corner reflectors, multiple faces, and provide helpful hints and a Building Reflection Utility to those wishing to try this out in their city.
Warning! The Sun's reflection is often bright enough to be enjoyed with eclipse glasses, implying that sunglasses are insufficient protection for your eyes. Further, consider the risk in damaging the camera's lens, sensor, and other internal parts before embarking on an extended imaging session.
This works perfectly well for the full Moon! Its reflected light is brighter than that shining from inside the building. Examples are shown below.
|

Figure 1. The Full Moon sets as the rising Sun reflects off windows. [A. Ling] |
Basics of a Single Reflection
|
Windows are far from optically flat, but barring exceptional designs and installations, the deviations average out to a plane surface. Hence we can apply Euclidean geometry:
- SAz is solar azimuth, BAz is building azimuth
-
β is the angle the building makes east of north
-
Formula also works when the view is from the west side.
-
For a reflection off the N or S face of a building in the E: SAz = 180 - BAz + 2*β
-
For a reflection off the N or S face of a building in the W: SAz = 540 - BAz + 2*β
Thanks to utilities/apps such as TPE (The Photographer's Ephemeris), it is now easy to obtain the building azimuth and the solar/lunar azimuths for any date/time. The building angle β can be estimated to almost 1 degree by using a protractor and Google maps, but just make sure you are measuring from overhead and not on a slant. Once you have confirmed a reflection, you can determine β exactly from the equations. Note that not all buildings are rectangular, so the adjacent or opposite face may be different than expected.
|
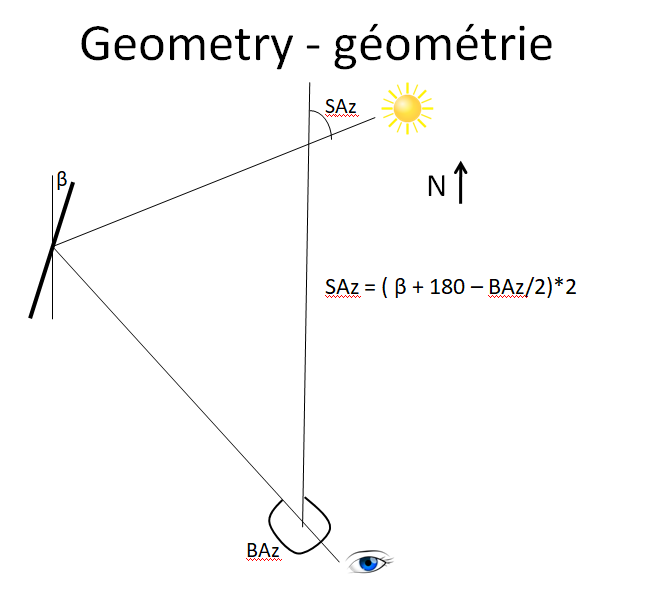
Figure 2. Plan view. |
A variant on the simple reflection is one of glancing incidence:

Figure 3. Glancing incidence from the building at right. [A. Ling] |
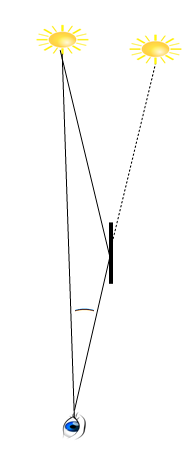
Figure 4. Schematic of glancing incidence. |
Corner reflectors
Corner reflectors (those with adjacent faces at 90 degrees) are found in protective clothing, on bicycles and road signs, right up to a cube left on the Moon's surface by the Apollo astronauts (used for precisely determining lunar distance). Their key property is that incident light is reflected directly back in the direction of the source, i.e. 180 degrees.
|
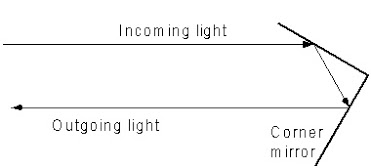
Figure 5. Corner reflector diagram from bikexprt.com
 Figure 6. The many corners of Manulife Tower [A. Ling] Figure 6. The many corners of Manulife Tower [A. Ling]
|
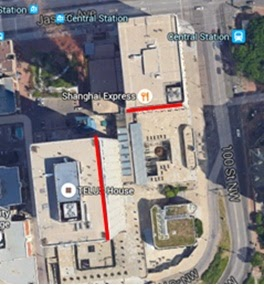 Figure 7. The faces may be on separate (detached) buildings[Google Maps] Figure 7. The faces may be on separate (detached) buildings[Google Maps] |
 Figure 8. An example with "classic" and detached corners [A. Ling] Figure 8. An example with "classic" and detached corners [A. Ling] |
Water: reflections in the vertical
|
At first you may not realize that capturing a water reflection scene often leads to a smaller Moon. Because you are including a mirror image, you typically have to double the vertical field of view, effectively halving the size of the Moon, and hence relinquishing the detail of its face. A 70mm lens on a cropped sensor camera has a field of roughly 15x10 degrees (landscape). The Moon climbs above 5 degrees pretty quickly, but shooting a wider field shrinks the image size just as quickly. The trade-off forces you to really plan ahead. Portrait framing mitigates this issue.
Another surprise may await. One expects to see a mirror image of the scene in the water, but as the water level drops farther below the horizon, the discrepancy between the direct and reflected image increases. Notice in Fig.9 that in the water, the Moon is about to be occulted by the building, but in the sky it is not even close. If you are standing right next to the water, the two would be very close to being mirror images. The view in Fig. 9 makes better sense physically if you imagine yourself floating on the water at the reflection point: from there, the building is "taller" (has a bigger angular height) because you are closer and additionally it is even higher in the sky because you are lower. The Moon would indeed be disappearing behind it. |

Figure 9. A reflected scene can mean accepting a smaller Moon [A. Ling] |
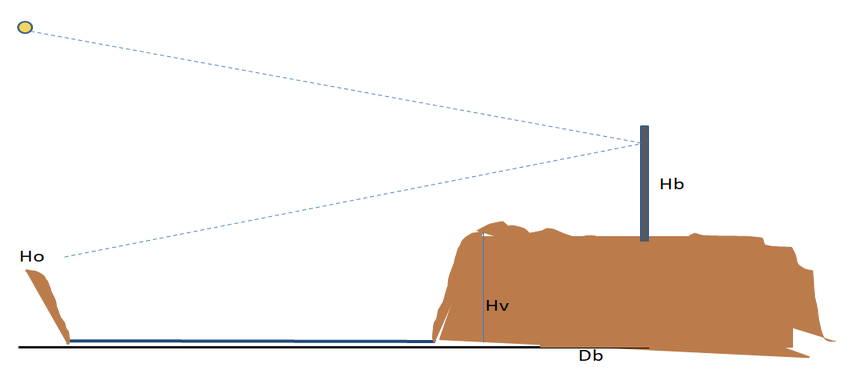 Figure 10. The geometry of a "standard" reflection in the vertical. Not to scale, the angles do not appear as they really are! Figure 10. The geometry of a "standard" reflection in the vertical. Not to scale, the angles do not appear as they really are!
- Hb: Height of the building above ground
- Db: Distance to the building
- Hv: Height of the valley above the water
- Ho: Height of the observer above the water
- Reflected Sun Alt (deg): atan ( [Hb+Hv-Ho]/Db) – 1/4
- Half the Moon's angular size is subtracted to get the full disk reflected
- Reduce the height of the building if windows do not go to the top
|

Figure 11. A "standard" reflection, with no water reflection [A. Ling] |
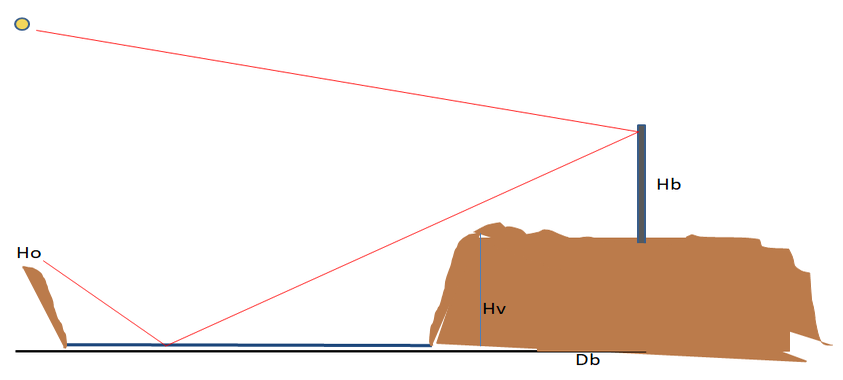 Figure 12. The geometry of a reflected reflection in the vertical Figure 12. The geometry of a reflected reflection in the vertical
- Reflected Sun Alt (deg): atan ( [Hb+Hv+Ho]/Db) - 1/4
- Half the angular size is subtracted to get the full disk reflected
- Reduce the height of the building if windows do not go to the top
- Distance to reflection point: Ho/tan(Alt)
- To see reflected in water: the Sun/Moon, so it is not occulted by the building: Alt: atan ( [Hb+Hv+Ho]/Db) + 1/4
|

Figure 13. A reflected reflection, with no "standard" reflection [A. Ling] |
The Azimuth and the Rise/Set Azimuth
After witnessing a sunrise reflection on one occasion, I eagerly calculated the orientation of a building's windows, looking forward to catch the event again a few weeks later from a different observation point based on the new sunrise azimuth. But the most that happened was a glow - the formula had failed! Once I ruled out inaccuracies in the angles provided by the software, curvature of the Earth, and errors in my geometry, I reexamined the particulars of the observing site: there was an intervening ridge occulting the bottom of the building, and I was closer, making the angular height of the building greater. The reflection had occurred, just below my line of sight.
Once I realized that the angular height of the reflecting windows had to be the same as the Sun, I then had the required Alt-Az pair which I could use for future events. A few months later we had an event far to the northwest on a taller building, altitude 2.7 degrees at azimuth 305.2. This reminded me that objects far to the north and south rise/set on much shallower angles than those near the equator. After fiddling around with the sunset date I found that the required set azimuth was 311.0, a difference of almost 6 degrees! In my list of "azimuths and altitudes for reflections" I added a column for the required rise/set azimuth using planetarium software. Despite the newfound success, I was annoyed by a discrepancy of about a half-degree. It turns out that The Photographer's Ephemeris and Guide use a different value for average refraction near the horizon, nicely resolving my issue.
A Web-based Reflection Utility
Once I had made a few finger blunders on the calculator and misclicks on the computer, I decided to create a web-based app. It sped up my corrections and eased the addition of three new towers in Edmonton to my list. Further, it is accessible to anyone wishing to try this for themselves:
http://www.nlcplanner.com/reflections/reflections.html.
Considerations and Further Subtleties
Lunar phase, multiple faces, non-90 degree angles, timing, atmospheric conditions, ripples on the water
Lunar phase, background light
If you are trying to catch the rising full Moon reflecting in a building, it typically needs to be more than 30 minutes after sunset, otherwise the background sky brightness detracts too much. The Moon's brightness drops significantly each day past full phase. At 3.5 days after, the reflections are not much brighter than the interior lights.
|
 Figure 14. Full Moon reflection 45 minutes after sunset [A. Ling] Figure 14. Full Moon reflection 45 minutes after sunset [A. Ling]
|

Figure 15. Reflections from a Moon 3.5 days past full. [A. Ling] |
Multiple Faces
When starting out, one tends to consider only direct and corner reflections. Now and then, due to luck, the unexpected happens, and you ask yourself "where did that reflection come from?" Using TPE's built-in Google maps and the formula above, you can quickly figure it out.
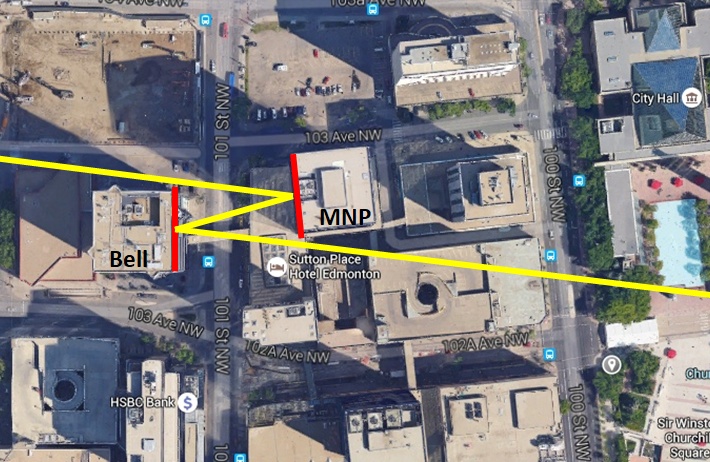 Figure 16. The geometry of a "double forward" reflection [Google Maps] Figure 16. The geometry of a "double forward" reflection [Google Maps] |

Figure 17. A "double forward" reflection [A. Ling] |
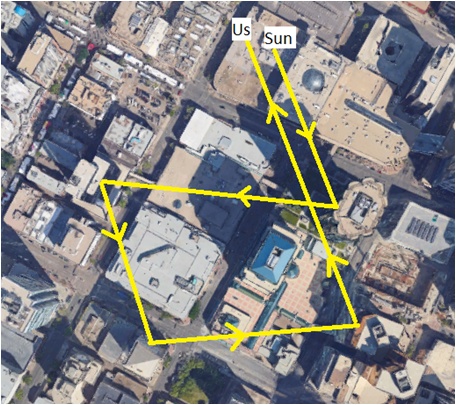 Figure 18. The geometry of a quadruple reflection [Google Maps] Figure 18. The geometry of a quadruple reflection [Google Maps] |

Figure 19. Orange flecks from a quadruple reflection [A. Ling]. The intensity has dropped significantly. |
Non-90 Degree Angles
|
 Figure 20. The geometry of a non-square corner reflection [Google Maps] Figure 20. The geometry of a non-square corner reflection [Google Maps]
- 2A = 180 + o - i
- Case 1) i is Sun (SAz – 90), o = ( BAz -270 )
SAz = BAz – 2A
- Case 2) i is building (BAz -270), o = (SAz – 90)
SAz = 2A + BAz - 360
|

Figure 21. Non-square angles on Edmonton's Pearl Tower [ Google Maps] |
Timing
Timing really is everything. On many occasions we got clouded out minutes before a rise or a set. On the other hand, we have been successful when the odds were against us - persistence is rewarded. Murphy's Law operates here: an ideal rise is thwarted by bad weather but the previous or following clear night the Moon's declination took it to a significantly different rise or set point, putting the buildings in the "wrong" place for a great composition. If you want to get a nice picture of the Moon above a well-lit foreground in a single exposure, it needs to be about 3 degrees above the horizon at sunset. 20 minutes can make or break a great opportunity. Much earlier and the sky will be too light, washing out the Moon. Much later and the sky and foreground will be too dark. The reverse occurs in the case of a moonset/sunrise paired event.
The "grail shot" is one with a nearly full Moon a few degrees above the horizon while the Sun is reflecting off the nearby buildings, as in Figure 1. If your city and its buildings are laid out on a roughly north-south oriented grid, as is Edmonton's, your opportunities for a grail capture are limited: you, the Moon, and the Sun need to be within a few degrees of due east/west for everything to line up. Due to the 8 to 10 degree displacement of the Moon in azimuth from one night to the next when it crosses the equator, the ideal geometry really comes only twice each equinox. Throw in bad weather, a rise or set 20 minutes earlier or later, a limited set of public observing locations, and a busy life, and you can see why it's not easy.
The inclination of the Moon's orbit to the ecliptic plays a role too. The rise/set points for an equinox full Moon shift back and forth with the Saros cycle, which may mean waiting 7 to 9 years for "the" alignment to recur.
Thankfully, many buildings and streets are not grid-locked; a 5 to 10 degree offset produces a wider window of opportunity.
Atmospheric Conditions
Just as with normal observing, weather plays a major factor in success or failure. The advantage of requiring only the last 2 degrees of sky at the horizon in the direction of the Sun or Moon to be clear is offset by foreshortening: a wide open door in front of you looks like a slit when viewed at a shallow angle. Experience points to needing about 3 to 4 degrees of longitude to be cloud-free in the appropriate direction. On cold humid days, the exhaust plumes from building power plants and industrial sites can readily block the Sun. Getting a wide clear band of longitude for a setting Moon and rising Sun (Fig. 1) is a lot tougher than you might think - all too often the cirrus shield from an advancing or retreating system is sufficient to knock down the light, but deep-sky observers could still have 5 hours of favourable skies in those cases.
Skywatchers are familiar with the belt of Venus, which is the pink-purple band of light capping the Earth's shadow opposite the Sun. For the evening case, it rises after sunset. We've been fooled a few times into thinking that the few clouds we saw before sunset have spread into a thick obscuring band only to be surprised and relieved to see the Moon rise unimpeded. Sometimes we do get clouded out, but the lesson is to not give up until you are sure it is too late: now and then a bit of cloud actually adds interest to the final composition.
The key effect of scattering is to modify brightness and colour. The Moon typically rises a deep orange and gradually transitions to pale yellow and eventually white. Unless you are doing HDR (High Dynamic Range, bracketed images), a single image can easily be under or over exposed. As long as your preview/review image reasonably captures the scene, you can rely on it for correctly exposing the Moon. If conditions look a little hazy, go for something slightly longer, then adjust to match the colour between real and digital as the event progresses. In very hazy conditions (eastern half of North America) typical of spring and summer, or air filled with forest fire smoke, you may not see the Moon (or Sun!) until it is well above the horizon.
For a solar reflection, thin, diffuse, or cirrus cloud reduces the intensity of the reflection but increases the duration of the glow on the building facade since it can easily double the apparent size of the Sun. This is a much greater problem for a lunar reflection, often resulting in a dull, unrecognisable glow.
Anomalous refraction occurs when the temperature profile is either strongly cold at the surface while warm aloft (an inversion), or undergoes more than one reversal. The primary impact is that the Sun (Moon) rises earlier or sets later by a couple of minutes, rarely several, which may cause a "miss" of a reflection.
Ripples
If the target, whether window or water, were optically flat, we would be able to see a nearly perfect reflection. For water, only the wavelet crests oriented in the right direction vertically can reflect the light back to the observer. Water waves are largely horizontal, so the reflection spreads in a vertical column as the range of wave slopes increases with the wind. Windows appear to have random amounts of warping, therefore the reflection spreads radially in all directions.
Conclusion
Observing solar and lunar reflections is enjoyable. I find their majestic pace to be relaxing, and it tickles one's fancy of anticipating the alignment of worlds. We may not have Stonehenge locally, but there is glasshenge. I hope the formulae and advice provided by this article enable you to easily seek out and observe these events.
References:
Ling, Alister Solar Reflections: How to Put Yourself in the Sunshine State
November 2005 The Journal of the Royal Astronomical Society of Canada. Royal Astronomical Society of Canada 99(6):205
Acknowledgments:
I would like to express my deep appreciation to Luca Vanzella for sharing this obsession with me. Not only do we inspire each other, the company makes for a safer session.





 Figure 6. The many corners of Manulife Tower [A. Ling]
Figure 6. The many corners of Manulife Tower [A. Ling] Figure 7. The faces may be on separate (detached) buildings[Google Maps]
Figure 7. The faces may be on separate (detached) buildings[Google Maps] Figure 8. An example with "classic" and detached corners [A. Ling]
Figure 8. An example with "classic" and detached corners [A. Ling]
 Figure 10. The geometry of a "standard" reflection in the vertical. Not to scale, the angles do not appear as they really are!
Figure 10. The geometry of a "standard" reflection in the vertical. Not to scale, the angles do not appear as they really are!

 Figure 12. The geometry of a reflected reflection in the vertical
Figure 12. The geometry of a reflected reflection in the vertical

 Figure 14. Full Moon reflection 45 minutes after sunset [A. Ling]
Figure 14. Full Moon reflection 45 minutes after sunset [A. Ling]
 Figure 16. The geometry of a "double forward" reflection [Google Maps]
Figure 16. The geometry of a "double forward" reflection [Google Maps]
 Figure 18. The geometry of a quadruple reflection [Google Maps]
Figure 18. The geometry of a quadruple reflection [Google Maps]
 Figure 20. The geometry of a non-square corner reflection [Google Maps]
Figure 20. The geometry of a non-square corner reflection [Google Maps]